In an earlier post, I wrote about how to measure heights using a mirror. However, in this short post, we will look at how to measure distances using a matchstick. In fact, any stick on which you can draw millimeter lines is suitable for this. This method is only applicable if we can roughly estimate the height of the object to which we are measuring the distance. Fortunately, for many objects, this is more or less known to us. For example, the average height of a person is 1.7 meters, and a car is 1.6 meters. If the object is located in a place inaccessible to us, for example behind a river, then its distance can be estimated very successfully using this simple method. Let's see how this distance estimation method works.
Using similar triangles
As when measuring heights with a mirror, we use the properties of similar triangles when measuring distances with a matchstick. Two triangles are similar if their corresponding angles are congruent and their corresponding sides are in equal proportion. Simply put, these triangles are the same shape but different sizes. For example, let's take a right triangle with side lengths of 3, 4, and 5 units.
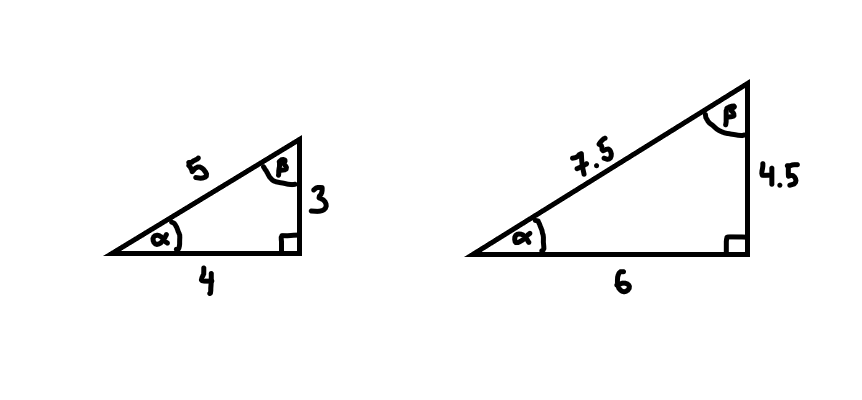
By increasing the side lengths of this triangle by 1.5 times, we get a new, larger triangle with side lengths of 4.5, 6, and 7.5 units. All the corresponding angles of these triangles are congruent, but the side lengths of the larger triangle are 1.5 times longer than the corresponding sides of the smaller triangle. One way to prove that triangles are similar is to make sure that the ratios of their corresponding sides are equal. If we take the ratios of the corresponding sides of our triangles, we see that they come out equal (6/4 = 4.5/3 = 7.5/5 = 1.5).
There are other ways to prove that triangles are similar. The easiest way is to make sure, that the corresponding angles of two triangles are congruent. Similarly, if the ratios of two pairs of corresponding sides of two triangles are equal and the included angles are congruent, then the triangles are similar.
A match as a simple range finder
Now let's make a simple distance meter. We take a match and draw lines with millimeter intervals on one side of it. Suppose you see a person across the river and want to determine the distance to him. Holding a match in an outstretched hand and looking with one eye, bring its upper end to such a position that it coincides with the upper end of a person in the distance.
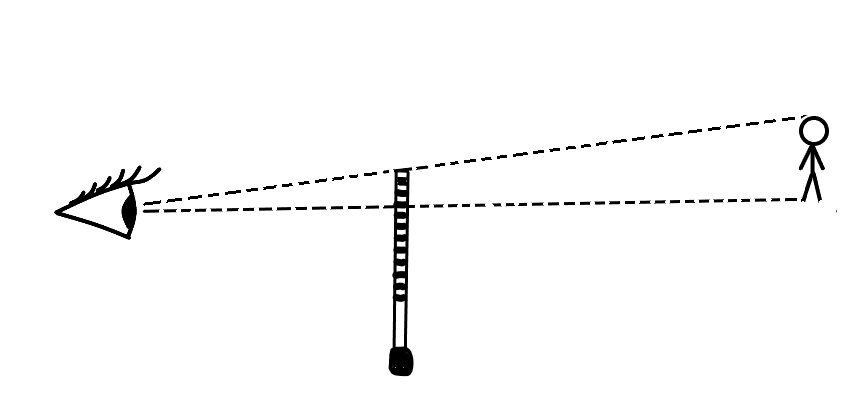
After that, slowly move your thumbnail up the match to the point where it aligns with the lowest point of the human figure. Now you have determined at which millimeter mark the thumbnail stopped. In the top image, the human figure in the distance appears to be 6 mm tall. Knowing approximately the distance of the eye from the match in the outstretched hand, we have all the necessary information to find the distance of the person beyond the river.
As you can see, two triangles are formed - triangle ABC and triangle ADE. Triangles ABC and ADE are similar because their corresponding angles are congruent.
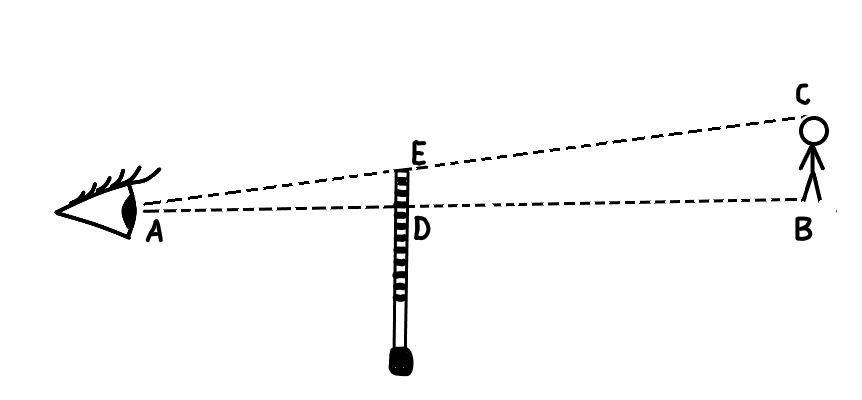
Using the property of similar triangles that the ratio of corresponding sides is equal, we can calculate the distance to the person across the river. The ratio of the average height of a person to the length measured on a match must be equal to the ratio of the unknown distance to the distance between the eye and the match.
Let's say, for example, that the distance between the eye and the match is AD=60 cm (600 mm), the height measured on the match is DE=6 mm, and the average height of a person is BC=170 cm (1700 mm).
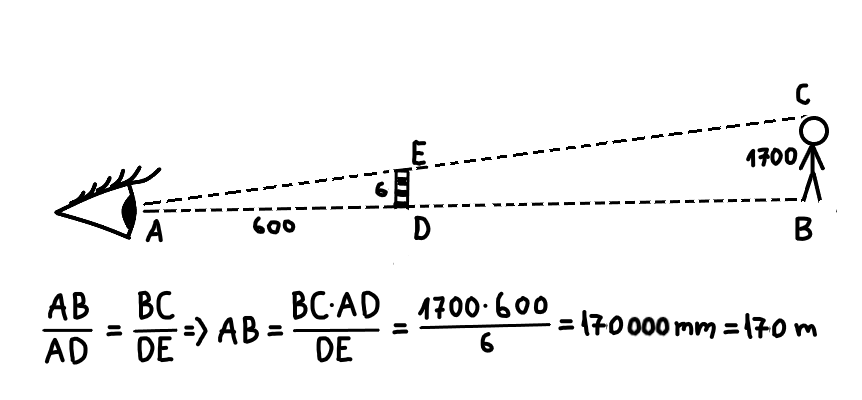
By writing the ratios of the corresponding sides (AB/AD=BC/DE), we can derive the unknown distance (AB) from the equation, which in our example is 170 meters.
This way of measuring the distances is not very accurate because we do not know precisely the heights of many objects. But for objects whose height we can roughly estimate, it can give a pretty good result.
留言